Sophomore Engineering Curricula	Introduction	Conservation and Accounting Framework	Curriculum Structure: Texas A&M four-course structure	Curriculum Structure: Texas A&M Five-Course Structure
	Curriculum Structure: Rose-Hulman Institute of Technology Sophomore Engineering Curriculum	Example Problems	Student Performance/Faculty Reactions	Conclusions

Example 1: Water Hammer

Consider a tank filled with water of constant density r. The tank has a constant area A and a pipe at the bottom from which a flow rate of Q exits. The pressure at the bottom of the tank is P. For demonstration purposes, the exiting flow rate is given. The problem could also be solved by expressing a relationship between the pressure at the tank bottom and flow rate. The problem is to derive an equation for the height of fluid in the tank, see Figure 1.

Figure 1 - Water tank with water exiting at a rate of Q.

The process requires the identification of the following:

1.      a boundary,

2.      properties to count,

3.      the degrees of freedom,

4.      the order.

If the water motion is “well behaved” with no turbulence or velocity profile, the model has a single degree of freedom. All the water moves together. If 1 unit of mass is removed, the position of all other mass is determinable. The following model will assume one dof.

The properties that can be counted include:

·        mass, which can be determined by the height of the fluid in the tank,

·        energy, which can be identified by fluid height and velocity,

·        momentum, accounted by fluid velocity.

Since fluid position and fluid velocity are independent, the system is at maximum, second order. The conditions that allow velocity to be neglected are small Q and large A.

Suppose the fluid speed is neglected. The model will be order one, degree of freedom one. Since the number of given motions is equal to the dof, the problem only requires expressing a kinematical constraint. That is what the conservation of mass does. The model consists of writing the conservation for the only “important” property, mass, and is:

                                  (1)

Now suppose the fluid speed is significant. The degree of freedom is still one, but the order is two. The mass conservation is identical to the previous but now the energy and momentum need to be handled. The conservation of linear momentum in the vertical direction can be easily derived.  First from the free body diagram shown in Figure 2 ,figure ?, the vertical force from the gage pressure at the bottom of the fluid is .

Figure 2 Freebody of the Water in the Tank.

The gravity force is . The momentum of the fluid in the tank is

(students sometimes miss the ½ because they forget that they are computing the velocity of the center of mass of the fluid). With these expressions, the conservation of linear momentum says:

                                      (2)

Now consider what these say. Suppose the flow rate is held constant for a while, the rate of change of h is a constant so the pressure at the tank bottom is equal to the fluid weight. Now suppose the flow rate is suddenly stopped. In other words,  at t^- and at t⁺, is discontinuous. When this happens equation 1 says (which was negative)  suddenly becomes zero, is discontinuous. This which means . Using this in the momentum equation (2) indicates that the pressure at the bottom of the tank suddenly jumps to for a very short time. Of course in the real world the pressure will not go to infinity because some of the fluid will leak, the container will expand slightly etc. but the pressure will be large. This large pressure for a short period is what is called water hammer. So what causes water hammer? Water hammer is caused by a sudden change in flow rate in a system where fluid momentum is not negligible.

What this example demonstrates is that (1) the conservation principles can derive the water hammer equations easily, (2) the use of degree of freedom helps to identify that the motion of the fluid height is directly related to the flow rate, (3) the use order helps to identify how many and what type of equations are required.

Before we leave this example, consider what conservation of momentum tells you in a system where the momentum and its change is insignificant. If the momentum and its change is insignificant, the term on the right of equation (2) is zero, hence: 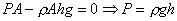 in other words, the pressure equals the static fluid weight (obviously). The point here is that the conservation equations are always valid and when written they will tell you something. Because of this point, some of the authors make a habit of teaching students to always write every conservation equation for every problem (this is an exaggeration of course but it makes the point). Order however is a useful tool to help determine the number and type of differential equations that are required for the model. The number and type of differential equations is important to know from a system dynamics or control point of view.

Back

References

1. Grinter, L.E. (Chair), Report on Evaluation of Engineering Education, American Society for Engineering Education, Washington, DC, 1955.
2.   Harris, Eugene M. DeLoatch, William R. Grogan, Irene C. Peden, and John R. Whinnery, "Journal of Engineering Education Round Table: Reflections on the Grinter Report," Journal of Engineering Education, Vol. 83, No. 1, pp. 69-94 (1994) (includes as an Appendix the Grinter Report, issued in September, 1955).
3. Glover, Charles, J., and Carl A. Erdman, "Overview of the Texas A&M/NSF Engineering Core Curriculum Development," Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992, pp. 363-367
4. Glover, Charles J., K. M. Lunsford, and John A. Fleming, “TAMU/NSF Engineering Core Curriculum Course 1: Conservation Principles in Engineering,” Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992, pp. 603-608
5. Glover, Charles J., K. M. Lunsford, and John A. Fleming, Conservation Principles and the Structure of Engineering, 3^rd edition, New York: McGraw-Hill College Custom Series, 1992
6. Pollock, Thomas C., “TAMU/NSF Engineering Core Curriculum Course 2: Properties of Matter,” Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992, pp. 609-613
7. Pollock, Thomas C., Properties of Matter, 3rd edition, New York: McGraw-Hill College Custom Series, 1992
8. Everett, Louis J., “TAMU/NSF Engineering Core Curriculum Course 3: Understanding Engineering via Conservation,” Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992, pp. 614-619
9. Everett, Louis J., Understanding Engineering Systems via Conservation, 2nd edition, New York: McGraw-Hill College Custom Series, 1992
10. Glover, Charles J. and H. L. Jones, “TAMU/NSF Engineering Core Curriculum Course 4: Conservation Principles for Continuous Media,” Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992 Conference, pp. 620-624
11. Glover, C. J. and H. L. Jones, Conservation Principles for Continuous Media, 2nd edition, New York: McGraw-Hill College Custom Series, 1992
12. Erdman, Carl A., Charles J. Glover, and V. L. Willson, “Curriculum Change: Acceptance and Dissemination,” Proceedings, 1992 Frontiers in Education Conference, Nashville, Tennessee, 11-14 November 1992, pp. 368-372
13. B. A. Black, “From Conservation to Kirchoff: Getting Started in Circuits with Conservation and Accounting,” Proceedings of the 1996 Frontiers in Education Conference, Salt Lake City, Utah, 6-9 November 1996
14. Griffin, Richard B., Louis J. Everett, P. Keating, Dimitris C. Lagoudas, E. Tebeaux, D. Parker, William Bassichis, and David Barrow, "Planning the Texas A&M University College of Engineering Sophomore Year Integrated Curriculum," Fourth World Conference on Engineering Education, St. Paul, Minnesota, October 1995, vol. 1, pp. 228-232.
15. Everett, Louis J., "Experiences in the Integrated Sophomore Year of the Foundation Coalition at Texas A&M," Proceedings, 1996 ASEE National Conference, Washington, DC, June 1996
16. Richards, Donald E., Gloria J. Rogers, "A New Sophomore Engineering Curriculum -- The First Year Experience," Proceedings, 1996 Frontiers in Education Conference, Salt Lake City, Utah, 6-9 November 1996
17. Heenan, William and Robert McLaughlan, "Development of an Integrated Sophomore Year Curriculum,” Proceedings of the 1996 Frontiers in Education Conference, Salt Lake City, Utah, 6-9 November 1996
18. Mashburn, Brent, Barry Monk, Robert Smith, Tan-Yu Lee, and Jon Bredeson, "Experiences with a New Engineering Sophomore Year,” Proceedings of the 1996 Frontiers in Education Conference, Salt Lake City, Utah, 6-9 November 1996
19. Everett, Louis J., "Dynamics as a Process, Helping Undergraduates Understand Design and Analysis of Dynamics Systems," Proceedings, 1997 ASEE National Conference,
20. Doering, E., “Electronics Lab Bench in a Laptop: Using Electronics Workbench to Enhance Learning in an Introductory Circuits Course,” Proceedings of the 1997 Frontiers in Education Conference, November 1997
21. Cornwell, P., and J. Fine, “Mechanics in the Rose-Hulman Foundation Coalition Sophomore Curriculum,” Proceedings of the Workshop on Reform of Undergraduate Mechanics Education, Penn State, 16-18 August 1998
22. Cornwell, P., and J. Fine, “Mechanics in the Rose-Hulman Foundation Coalition Sophomore Curriculum,” to appear in the International Journal of Engineering Education
23. Cornwell, P. and J. Fine, “Integrating Dynamics throughout the Sophomore Year,” Proceeedings, 1999 ASEE Annual Conference, Charlotte, North Carolina, 20-23 June 1999
24. Burkhardt, H. "System physics: A uniform approach to the branches of classical physics." Am. J. Phys. 55 (4), April 1987, pp. 344–350.
25. Fuchs, Hans U. Dynamics of Heat. Springer-Verlag, New York, 1996.

Back